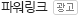Let P1, P2, ... , P8 be 8 distinct points on a circle. Determine the number of possible configurations
made by drawing a set of line segments connecting pairs of these 8 points, such that : (1) each Pi is
the endpoint of at most one segment and (2) two no segments intersect. (The configuration with no
edges drawn is allowed. An example of a valid configuration is shown below.)мЈ„мҶЎн•©лӢҲлӢӨ.. м ңк°Җ к·ёлҰјмқ„ мһҳ лӘ»к·ёл Өм„ң;; м Җкұ° мӣҗмқҙкі мҡ” к°Ғ м җмқҖ 8л“ұ분м җмқҙкі м„ лҸ„ м§Ғм„ мқҙмҳҲмҡ”
мқҢ;; м ңк°Җ м—¬кё°м„ңк¶ҒкёҲн•ңкұҙмҡ” 2м§ёмӨ„м—җ a set of line segments лқјкі лӮҳмҷҖмһҲлҠ”лҚ° segmentк°Җ ліөмҲҳлЎң лӮҳмҷ”лӢӨлҠ”кұҙ
н•ңмӨ„л§Ң м—°кІ°н•ң кІҪмҡ°мқҳ мҲҳлҠ” л№јлқјлҠ” лң»мқёк°Җмҡ”? м•„лӢҲл©ҙ м җмқҙ 2к°ңмқҙмғҒ л”°лЎң лӮЁм•„мһҲм§Җ м•ҠкІҢн•ҳлқјлҠ”кұҙк°Җмҡ”?
мҳҲлҘј л“Өм–ҙ л‘җмӨ„мқ„ м—°кІ°н•ңлӢӨл©ҙ м–ҙл–Ө л°©лІ•мңјлЎң м—°кІ°мқ„ н•ҳлҚ”лқјлҸ„ н•ңлІҲлҚ” м—°кІ°н• мҲҳ мһҲмһ–м•„мҡ”
к·ёлҹј мӨ„3к°ңлҘј мқҙмқ„ л°©лІ• + мӨ„4к°ңлҘј мқҙмқ„л°©лІ• мқ„ кө¬н•ҳлқјлҠ” л¬ём ңмқёк°Җмҡ”?
м•„лӢҳ мӨ„1к°ңлҘј мқҙмқ„ л°©лІ•л¶Җн„° мӨ„4к°ңлҘј мқҙмқ„ л°©лІ•к№Ңм§Җ лӘЁл“ кІҪмҡ°мқҳмҲҳлҘј кө¬н•ҳлқјлҠ” л¬ём ңмқёк°Җмҡ”?
2022-02-18 01:36:49
мЈјмҶҢ : м„ңмҡёмӢң к°•м„ңкө¬ нҷ”кіЎлҸҷ 786-9 нғ‘кұҙ 302нҳё | л¬ёмқҳ : kjs8907@nate.com